The Mathematical Process Standards are skills in the Texas Essential Knowledge and Skills (TEKS) which describe ways students are expected to engage with the content. These standards should be incorporated into the teaching of the TEKS when possible so that students can attain a greater depth of understanding of complex content. The process standards are integrated at every grade level and course. When embedded meaningfully in the classroom, these process standards can provide a rich learning environment to help students make sense of mathematics in the real world.
To help teachers successfully embed these process standards in everyday instruction, we took each standard and followed it with a bulleted list of actions we expect mathematically proficient students to exhibit. Each bulleted list is followed by a short description of how teachers can effectively incorporate each process standard in the classroom.
Additionally, we believe these mathematical process standards can be made more impactful if the text is interpreted in terms of behaviors one should expect to observe as students engage with each process standard. Ideally, students would view the bulleted statements as the success criteria by which they could self-assess their ability to become successful problem solvers and use mathematics efficiently and effectively in daily life.
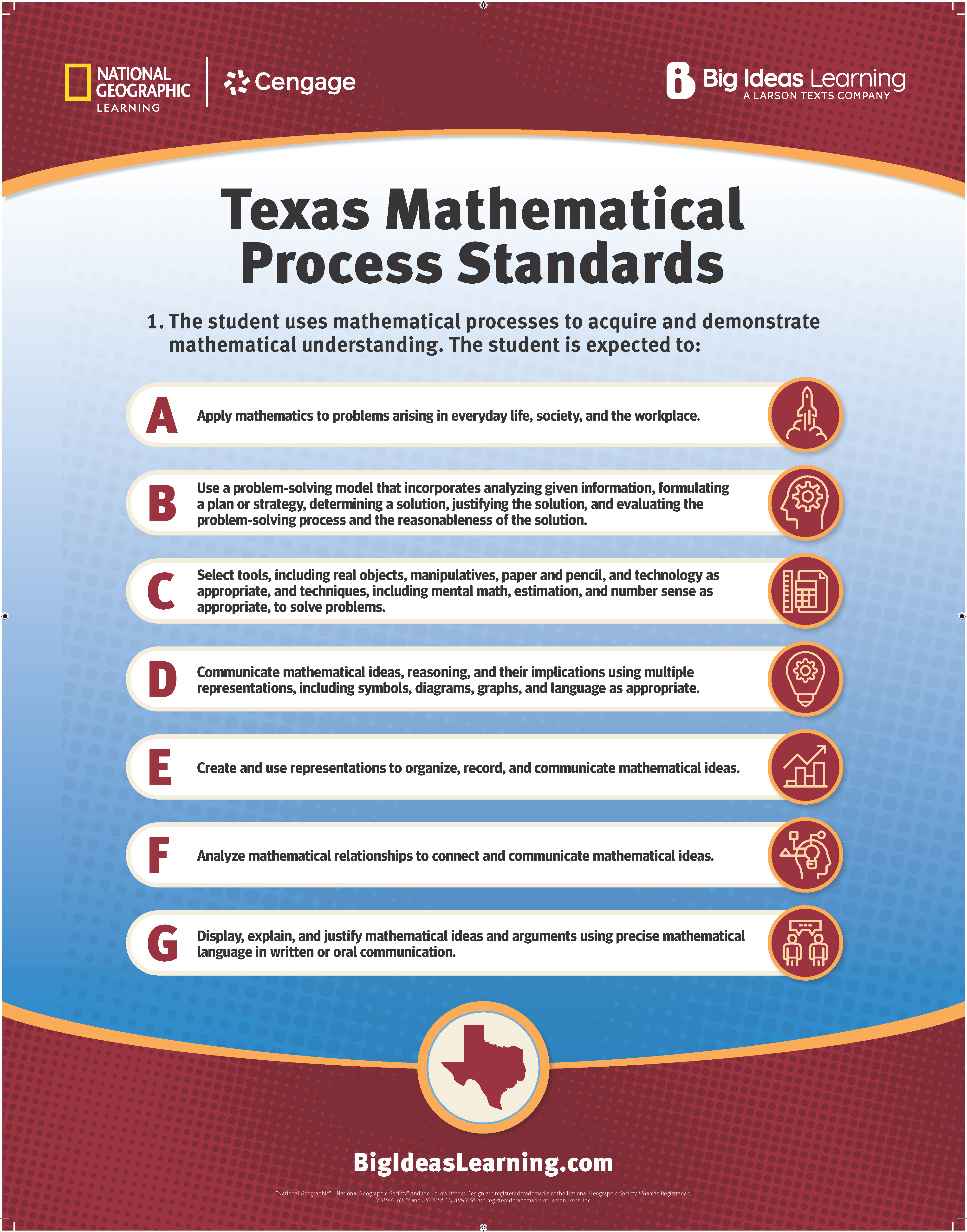
(1) Mathematical process standards. The student uses mathematical processes to acquire and demonstrate mathematical understanding. The student is expected to:
(A): APPLY MATHEMATICS TO PROBLEMS ARISING IN EVERYDAY LIFE, SOCIETY, AND THE WORKPLACE;
Mathematically proficient students:
- Connect mathematical concepts to everyday experiences.
- Explain the meaning of a problem and look for entry points to its solution.
- Analyze givens, constraints, relationships, and goals.
- Plan a solution pathway rather than simply jumping into a solution.
- Use concrete objects or pictures to help conceptualize and solve a problem.
- Use models and methods to understand, represent and solve problems.
- Redesign models and methods to improve accuracy or efficiency.
To incorporate process standard (A) effectively in the classroom, teachers can help students by first cultivating a community of growth mindset learners. They can help students connect mathematical concepts to everyday experiences by choosing tasks that are both interesting and challenging. Teachers should look to present problems that allow for multiple strategies and multiple solutions. Importantly, teachers should recognize students’ efforts when solving challenging problems.
(B): USE A PROBLEM-SOLVING MODEL THAT INCORPORATES ANALYZING GIVEN INFORMATION, FORMULATING A PLAN OR STRATEGY, DETERMINING A SOLUTION, JUSTIFYING THE SOLUTION, AND EVALUATING THE PROBLEM-SOLVING PROCESS AND THE REASONABLENESS OF THE SOLUTION;
Mathematically proficient students:
- Analyze the problem in a way that makes sense given the task.
- Create a plan for solving the problem.
- Continually ask, “Is this reasonable?” when working through a task.
- Apply prior knowledge to independently model, understand, and represent real-world problems.
- Identify, analyze, and draw conclusions about quantities using tools such as diagrams, two-way tables, graphs, flowcharts, and formulas.
- Check to see if an answer makes sense within the context of a situation, possibly improving/revising the model.
- Evaluate the problem-solving process and look for improving efficiency for similar tasks where possible.
To incorporate process standard (B) effectively in the classroom, teachers should provide opportunities for students to create models, both concrete and abstract, and perform investigations. They should ask students to justify their choice of model and the thinking behind it, as well as the appropriateness of the model chosen. Teachers can also assist students in seeing and making connections among different models.
(C): SELECT TOOLS, INCLUDING REAL OBJECTS, MANIPULATIVES, PAPER AND PENCIL, AND TECHNOLOGY AS APPROPRIATE, AND TECHNIQUES, INCLUDING MENTAL MATH, ESTIMATION, AND NUMBER SENSE AS APPROPRIATE, TO SOLVE PROBLEMS;
Mathematically proficient students:
- Make good decisions about which tools to use and how to use the tools in specific situations.
- Use technological tools to visualize, analyze, and help solve problems, and to deepen math knowledge.
- Identify and use external math resources to pose and solve problems.
- Use estimation and other mathematical knowledge to detect possible errors.
To incorporate process standard (C) effectively in the classroom, teachers should help students see why the use of manipulatives, rulers, compasses, protractors, calculators, statistical software, and other tools will aid their problem-solving processes. They should make sure that math tools and manipulatives are readily available and frequently model the use of appropriate tools. Teachers should give students a choice of materials and tools and have discussions with them about their choices to lead them to use appropriate tools strategically.
(D): COMMUNICATE MATHEMATICAL IDEAS, REASONING, AND THEIR IMPLICATIONS USING MULTIPLE REPRESENTATIONS, INCLUDING SYMBOLS, DIAGRAMS, GRAPHS, AND LANGUAGE AS APPROPRIATE;
Mathematically proficient students:
- Communicate mathematical ideas, vocabulary, and methods effectively.
- Represent solutions to problems in multiple ways using objects, drawings, tables, graphs, and equations.
- Flexibly move between concrete and abstract representations of problem situations.
- Express connections between concepts and representations.
- Choose a representation based on the given context or purpose.
To incorporate process standard (D) effectively in the classroom, teachers should establish a culture in which students ask questions of the teacher and their peers, and communicate their reasoning behind choosing particular representations of problems. Teachers should support mathematical discussions that probe and extend students’ understanding. They can guide students from concrete to pictorial to abstract representations as understanding progresses, and expect students to give meaning to all quantities in a task. Additionally, teachers can strategically sequence and use student responses to highlight mathematical ideas and language.
(E) CREATE AND USE REPRESENTATION TO ORGANIZE, RECORD, AND COMMUNICATE MATHEMATICAL IDEAS;
Mathematically proficient students:
- Draw diagrams of important features and relationships, record and graph data, and search for regularity or trends.
- Explain correspondences between verbal descriptions, tables, graphs, and equations.
- Interpret mathematical ideas presented by various representations.
- Communicate mathematical ideas by various presentations effectively.
- Are careful about the meaning of units and clearly and accurately label diagrams.
To incorporate process standard (E) effectively in the classroom, teachers should encourage students to represent their ideas in ways that make sense to them, even if their first representations are not conventional ones. Teachers can help students connect their personal representations to more-conventional ones, when appropriate. It is important that students have opportunities not only to learn conventional forms of representation but also to construct, refine, and use their own representations as tools to support learning and doing mathematics.
(F): ANALYZE MATHEMATICAL RELATIONSHIPS TO CONNECT AND COMMUNICATE MATHEMATICAL IDEAS;
Mathematically proficient students:
- Analyze and evaluate the mathematical thinking and strategies of others.
- Understand how mathematical ideas interconnect and build on one another to produce a coherent whole.
- Recognize and apply mathematics in contexts outside of mathematics.
- Communicate mathematical thinking coherently and clearly to peers, teachers, and others.
To incorporate process standard (F) effectively in the classroom, teachers should look to present real-world problems that allow for multiple strategies and multiple solutions. They should ask students to share their strategies and thinking with each other, while looking for ways in which their ideas interconnect. Specifically, ask “how, why, and when” questions to prompt students to reflect on their reasoning.
(G): DISPLAY, EXPLAIN, AND JUSTIFY MATHEMATICAL IDEAS AND ARGUMENTS USING PRECISE MATHEMATICAL LANGUAGE IN WRITTEN OR ORAL COMMUNICATION;
Mathematically proficient students:
- Use clear and precise definitions in discussion with others and in their own reasoning.
- Construct possible arguments based on stated assumptions, definitions, and previously established results.
- Analyze the arguments of others and ask probing questions to clarify or improve the arguments.
- Compare the effectiveness and efficiency of two or more plausible arguments.
- Justify results by explaining mathematical ideas, vocabulary, and methods effectively.
To incorporate process standard (G) effectively in the classroom, teachers should consistently model the use of precise mathematical language and symbols and expect their students to do the same. They should create opportunities for students to discuss their thinking with peers and help develop students’ ability to justify methods and compare their responses to the responses of their peers. Teachers should also establish a habit of selecting, sequencing and presenting student work to advance and deepen understanding of correct and increasingly efficient methods.
In closing, we believe the goal should be to engage students in rich, high-level mathematical tasks that support the approaches, practices, and habits of mind which are called for within these process standards.


